| 数学是不是先验的? |
| 送交者: 直言 2012年12月13日12:10:47 于 [五 味 斋] 发送悄悄话 |
|
来五味匆匆一瞥,惊见如下对话:
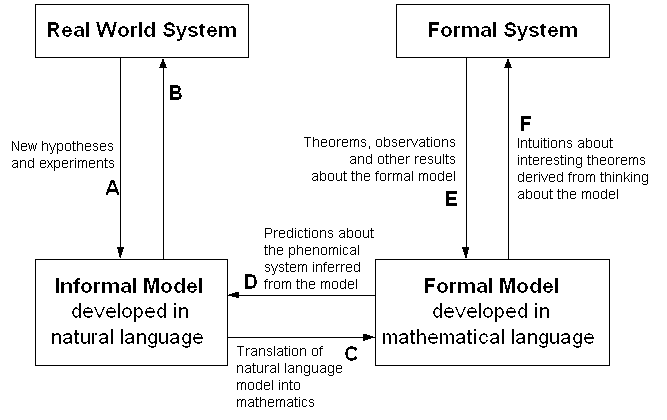
紫荆棘鸟:逻辑基本上是先验的,数学不是。三段论本身不会有差池。 (http://bbs.creaders.net/life/bbsviewer.php?btrd_id=2615709&btrd_trd_id=802925) 阿拉无语。历时数分钟,方缓缓回过神来。 首先,顶禽兽同学这句话:”阿拉比较欣赏能把复杂问题很简单地说给文盲听“。 禽兽同学不喜欢长篇大论,却常常于语不惊人的短语中发出令人眩目的火花,是五味为数不多的几位具备大智慧的同学,与五味几位运算上的巨人、逻辑上的侏儒、梦中的真理灯塔、现实中的盲人的同学形成鲜明对比。 本人一贯灰强敬仰。 另外,禽兽同学“如果你指欧式几何vs非欧几何,我同意”不仅正确,而且讲得灰强到点。 数学是否先验,是个认识论范畴的问题。了解或者明白人类对这个问题认知上的进展,毫无晦涩难懂之处,既无任何神秘,也不需要多么高深的知识或特殊的抽象思维能力,灰强简单,有基本阅读能力和康门三四即可理解明白。 简单地回答,就是,数学是先验的古典哲学(以康德为代表)观点在近代受到了挑战。如此而已。 下面推荐一篇综述,简明扼要地介绍了这一过程,有基本英文阅读能力的同学皆可以读懂: Mathematics is the model of a-priori knowledge in the Aristotelian tradition of rationalism. Among the Greeks, geometry was regarded as the highest form of knowledge, a potent key to the metaphysical mysteries of the Universe. This is a rather mystical belief, and the connection to mysticism and religion was made explicit in cults like the Pythagorean. No culture since has semi-deified a man for discovering a geometrical theorem! The Greek awe of mathematical knowledge is still with us; it's behind the traditional metaphor of mathematics as "Queen of the Sciences". It's been reinforced by the spectacular successes of mathematical models in science, successes the Greeks (lacking even simple algebra) could never have foreseen. Since Isaac Newton's discovery of calculus and the inverse-square law of gravity in the late 1600s, phenomenal science and higher mathematics have been closely symbiotic -- so much so, that the existence of a predictive mathematical formalism has become the hallmark of a "hard science". For two centuries after Newton, phenomenal science aspired to the kind of rigor and purity that seemed to be embodied in mathematics. The metaphysical situation seemed simple; mathematics embodied perfect a-priori knowledge, those sciences able to most mathematicize themselves were the most successful at phenomenal prediction; perfect knowledge would therefore consist of a mathematical formalism, arrived at by science and embracing all of reality, that would ground a-posteriori empirical understanding in a-priori rational logic. It was in this spirit that Condorcet dared to imagine describing the entire universe as a mutually-solving set of partial differential equations. The first cracks in this inspiring picture appeared in the latter half of the 19th century when Riemann and Lobachevsky independently proved that Euclid's Axiom of Parallels could be replaced by alternatives which yielded consistent geometries. Riemann's geometry was modeled on a sphere, Lobachevsky's on a hyperboloid of rotation. The impact of this discovery has been obscured by later and greater upheavals, but at the time it broke on the intellectual world like a thunderbolt. For the existence of mutually inconsistent axiom systems for geometry, any of which could be modeled in the phenomenal universe, called the whole relationship between mathematics and physical theory into question. When there was only Euclid, there was only one possible geometry. One could believe that the Euclidean axioms constituted a kind of perfect a-priori knowledge about geometry in the phenomenal world. But suddenly we had three geometries, an embarrassment of metaphysical riches. For how were we to choose between the axioms of plane, spherical, and hyperbolic geometry as a description of "real" geometry? Because all three are consistent, we couldn't choose on any a-priori basis -- the choice had to become empirical, based on their predictive power for a given situation. Of course, physical theorists had long been accustomed to choosing formalisms to fit a scientific problem. But it had been widely, if unconsciously, assumed that the need to do so ad hoc was a function of human ignorance; that, given good enough mathematics and logic, we could deduce the correct choice from first principles, producing a-priori descriptions of reality to be confirmed, as an afterthought, by empirical check. But now, the Euclidean geometry that had been considered the model for axiomatic perfection in mathematics for over two thousand years, had been dethroned. If one could not know something as fundamental as the geometry of space a-priori, what hope was there for a purely "rational" theory encompassing all of nature? Psychologically, Riemann/Lobachevsky struck at the very heart of the enterprise of mathematics as it was then conceived. Furthermore, Riemann/Lobachevsky called the nature of mathematical intuition into question. It had been easy to believe implicitly that mathematical intuition was a form of perception -- a glimpse of the Platonic noumena behind reality. But with two other geometries jostling Euclid, nobody knew for sure what the noumena looked like any more! Mathematicians responded to this dual problem with an increase in rigor, by trying to apply the axiomatic method throughout mathematics. It was gradually realized that the belief in mathematical intuition as a kind of perception of a noumenal world had encouraged sloppiness; proofs in the pre-axiomatic period often relied on shared intuitions about mathematical "reality" that could no longer be considered automatically valid. The new thinking in mathematics led to a series of spectacular successes; among these were Cantorian set theory, Frege's axiomatization of number, and eventually Russell & Whitehead's monumental synthesis in Principia Mathematica. However, it also had a price. The axiomatic method made the connection between mathematics and phenomenal reality narrower and narrower. At the same time, discoveries like the Banach-Tarski Paradox suggested that mathematical axioms that seemed to be consistent with phenomenal experience could lead to dizzying contradictions with that experience. The majority of mathematicians quickly became "Formalists", holding that pure mathematics could not be philosophically considered more than a sort of elaborate game played with marks on paper (this is the theory behind Robert Heinlein's pithy characterization of mathematics as "a zero-content system"). The old-fashioned "Platonist" belief in the noumenal reality of mathematical objects seemed headed for the dustbin, despite the fact that mathematicians continued to feel like Platonists during the process of mathematical discovery. Philosophically, then, the axiomatic method lead most mathematicians to abandon previous beliefs in the metaphysical specialness of mathematics. It also created today's split between pure and applied mathematics. Most of the great mathematicians of the early modern period -- Newton, Liebniz, Fourier, Gauss, and others -- were also phenomenal scientists (i.e. "natural philosophers"). The axiomatic method incubated the modern idea of the pure mathematician as super-esthete, unconcerned with the merely physical Ironically, Formalism gave pure mathematicians a bad case of Platonic attitude. Applied mathematicians stopped being invited to tea and learned to hang out with physicists. This brings us to the early 20th century. For the beleaguered minority of Platonists, worse was yet to come. Cantor, Frege, Russell and Whitehead showed that all of pure mathematics could be built on the single axiomatic foundation of set theory. This suited the Formalists just fine; mathematics coalesced, at least in principle, from a bunch of little disconnected games to one big game. It also made the Platonist minority happy; if there turned out to be one big, over-arching consistent structure behind all of mathematics, the metaphysical specialness of mathematics might yet be rescued. Unfortunately, it turns out that there is more than one way to axiomatize set theory. In particular, there are at least four major different combinations of assumptions about infinite sets that lead to mutually exclusive set theories (the Axiom of Choice or its negation; the Continuum Hypothesis or its negation). It was Riemmann/Lobachevsky all over again, but on a much more fundamental level. Riemannian and Lobachevskian geometry could be modeled finitely, in the world; you could decide at least empirically which one fit. Normally, you could regard all three as special cases of the geometry of geodesics on manifolds, thereby fitting them into the superstructure erected on set theory. But the independent axioms in set theory don't seem to lead to any results that can be modeled in the observable finite world. And there's no way to assert both the Continuum Hypothesis and its negation in one set theory. How's a poor Platonist to choose which system describes "real" mathematics? The victory of the Formalist position seemed complete. In a negative way, though, a Platonist had the last laugh. Kurt Godel threw a spanner in the Formalist program of axiomatization when he showed that any axiom system powerful enough to include the integers would have to be either inconsistent (yielding contradictions) or incomplete (too weak to decide the truth or falsehood of some assertions in the system). And that is more or less where things stand today. Mathematicians know that any attempt to put forward mathematics as a-priori knowledge about the universe must fall afoul of numerous paradoxes and impossible choices about what axiom system describes "real" mathematics. They've been reduced to hoping that the standard axiomatizations are not inconsistent but incomplete, and wondering uneasily what contradictions or unprovable theorems are waiting discovery out there, lurking like landmines in the noosphere. Meanwhile, on the empirical front, mathematics continued to be a spectacular success as a theory-building tool. The great successes of 20th-century physics (general relativity and quantum mechanics) wandered so far from the realm of physical intuition that they could be understood only by meditating deeply on their mathematical formalisms, and following through to their logical conclusions, even when those conclusions seem wildly bizarre. What irony. Even as mathematical 'perception' came to seem less and less reliable in pure mathematics, it became more and more indispensable in phenomenal science! Against this background, Einstein's famous quote wondering at the applicability of mathematics to phenomenal science poses an even thornier problem than at first appears. The relationship between mathematical models and phenomenal prediction is complicated, not just in practice but in principle. Much more complicated because, as we now know, there are mutually exclusive ways to axiomatize mathematics! It can be diagrammed as follows (thanks to Jesse Perry for supplying the original of this chart):
We begin to get some handle on the problem if we phrase it more precisely; that is, "Why does a good choice of C so often yield new knowledge via D?" The simplest answer is to invert the question and treat it as a definition. A "good choice of C" is one which leads to new predictions. The choice of C is not one that can be made a-priori; one has to choose, empirically, a mapping between real and mathematical objects, then evaluate that mapping by seeing if it predicts well. For example, the positive integers are a good formalism for counting marbles. We can confidently predict that if we put two marbles in a jar, and then put three marbles in a jar, and then empirically associate the set of two marbles with the mathematical entity 2, and likewise associate the set of three marbles with the mathematical entity 3, and then assume that physical aggregation is modeled by +, then the number of marbles in the jar will correspond to the mathematical entity 5. The above may seem to be a remarkable amount of pedantry to load on an obvious association, one we normally make without having to think about it. But remember that small children have to learn to count...and consider how the above would fail if we were putting into the jar, not marbles, but lumps of mud or volumes of gas! One can argue that it only makes sense to marvel at the utility of mathematics if one assumes that C for any phenomenal system is an a-priori given. But we've seen that it is not. A physicist who marvels at the applicability of mathematics has forgotten or ignored the complexity of C; he is really being puzzled at the human ability to choose appropriate mathematical models empirically. By reformulating the question this way, we've slain half the dragon. Human beings are clever, persistent apes who like to play with ideas. If a mathematical formalism can be found to fit a phenomenal system, some human will eventually find it. And the discovery will come to look "inevitable" because those who tried and failed will generally be forgotten. But there is a deeper question behind this: why do good choices of mathematical model exist at all? That is, why is there any mathematical formalism for, say, quantum mechanics which is so productive that it actually predicts the discovery of observable new particles? The way to "answer" this question is by observing that it, too, properly serves as a kind of definition. There are many phenomenal systems for which no such exact predictive formalism has been found, nor for which one seems likely. Poets like to mumble about the human heart, but more mundane examples are available. The weather, or the behavior of any economy larger than village size, for example -- systems so chaotically interdependent that exact prediction is effectively impossible (not just in fact but in principle). There are many things for which mathematical modeling leads at best to fuzzy, contingent, statistical results and never successfully predicts 'new entities' at all. In fact, such systems are the rule, not the exception. So the proper answer to the question "Why is mathematics is so marvelously applicable to my science?" is simply "Because that's the kind of science you've chosen to study!" |
|
|
|
|
 |
 |
| 实用资讯 | |
|
|
|
|
| 一周点击热帖 | 更多>> |
|
|
|
| 一周回复热帖 |
|
|
|
|
| 历史上的今天:回复热帖 |
| 2011: | 俺在小学选举过班长 | |
| 2011: | 4个东北人在南方绞杀10个小姐的思想 | |
| 2010: | 哈哈,我胡汉三又回来了 | |
| 2010: | 我真不敢相信怎么能让那个到荷兰大使馆 | |
| 2009: | 从杨元元自杀,可以看出中国穷人的路越 | |
| 2009: | 靠,陪着小老大把蜗居后面几集看完了 | |
| 2008: | 说说看,在国外生活一般要用多长时间才 | |
| 2008: | 贴一革命歌曲(外一首) | |
| 2007: | 我给大家先讲一个贵族的故事,嘿嘿 | |
| 2007: | 讲一个看来的故事 | |